09 Wave Phenomena

$y$ is half the width of the central maxima
$L$ is the distance between the slit and the screen

Diffraction

Interference

Diffraction grating

Increase in
slit number

Primary maxima

Secondary maxima

Increased intensity

More maxima ( $N-2$ )

Thinner width

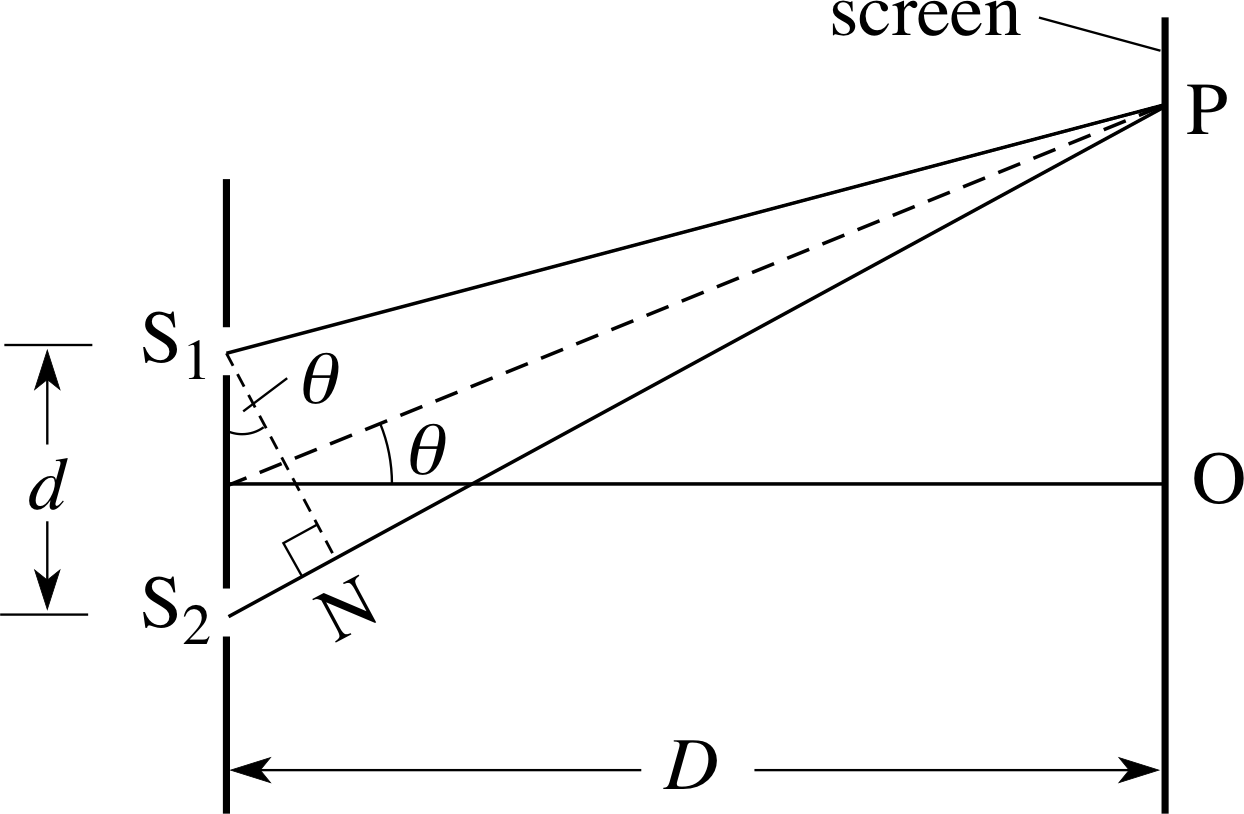
$S_2P-S_1P=n\lambda$
If $D>d,$
$\sin\theta=\frac{n\lambda}{d}$
$\tan\theta=\frac{X_n}{D}$

If theta is small, $\tan\theta=\sin\theta$
$\frac{X_n}{D}=\frac{n\lambda}{d}$
$X_n=\frac{n\lambda D}{2}$

Resolution

Resolving Power

Questions

Nov 2016

Q5 (Interference Patterns)

Q6 (Doppler Effect, Resolution)

Nov 2017

Q6 (Slits)

Nov 2018

Q4c (Interference Pattern)

Q5b (Diffraction)

Conditions

Good diffraction 👍

$\lambda ≥ b$

$\lambda$ is the wavelength
$b$ is the size of the aperture

Poor diffraction 👎

$\lambda\ll b$

Single-Slit

dropped image link

Angle of first minimum

Since its FIRST minimum

$P.D.=\frac{n\lambda}{2}$
n=1

$\frac{d}{2}\sin\theta=\frac{\lambda}{2}$

$d\sin\theta=\lambda$

$\theta\ (rads)=\frac{\lambda}{d}$

Every light ray on top corresponds to a ray below with path difference x

Length L

$y$ is half the width of the central maxima
$L$ is the distance between the slit and the screen

$\tan\theta=\frac{y}{L}$

Huygens' principle
Every point on wavefront that hits slit becomes a soucrce of spherical secondary wavelets

Double Slit

dropped image link

Linear Separation
between maxima

$s_n=D\tan\theta$

$s_n$

$D$: distance from screen
$s_n$: distance between maxima

$s_n=D\sin\theta$

$s_n=\frac{Dn\lambda}{d}$

$\tan\theta\approx\sin\theta$

$d\sin\theta=n\lambda$

Inductive Process
$s=\frac{D\lambda}{d}$

Contains a large number of parallel and closely spaced slits

Less bright

NOT IN FORMULA BOOKLET

Increase in slit
separation

Interference due to

interference of light from two slits

Interference of light from single slit

$d\sin\theta =n\lambda;\ n =0,1,2,…$

Thin film Interference

When expressed as rulings per mm
$d=\frac{1}{N}\times10^{-3}m$

dropped image link

Phase change (A) of $\mathbf{\pi}$ radians when:
Optically less dense (low refractive index) REFLECTS OFF
Optically denser (high refractive index)

No phase change (B) when:
Optically denser (high refractive index)
REFLECTS OFF
Optically less dense (low refractive index)

Assuming angle of incidence is small

$\textrm{Path Difference}=2d$

Destructive Interference

Constructive Interference

One phase change

$n$ is refractive index of other medium

$2dn=(m+\frac{1}{2})\lambda_{oil}$

No / Two phase change

$2dn=m\lambda$

Opposite

$2dn=(m+\frac{1}{2})\lambda$

$\lambda_{oil}=\frac{\lambda}{n}$

Increase in d
Decrease in $\sin\theta$

Light

Coherent

Monochromatic

Different light rays have constant phase difference

Of a single wavelength

$R=\frac{\lambda_{avg}}{\triangle\lambda}$

Rayleigh Criterion

Central maximum of diffraction pattern is aligned with the first minimum of the other diffraction pattern

Separation angle ≥ Diffraction angle
$\theta_S≥\theta_D$

Angle of 1st Minima $\theta_D$

Single Slit

$\theta=\frac{\lambda}{b}$

Circular Slit

dropped image link

$\theta=1.22\frac{\lambda}{b}$

Separation angle $\theta_s$

dropped image link

$\theta\ (rads)=\frac{s}{d}$

$s$ is distance between sources
$d$ is distance from source to observer

$R=mN$

Smallest resolvable difference

$\Delta\lambda=\frac{\lambda_{avg}}{mN}$

Doppler Effect

Moving Source

Away

Moving Observer

Towards

$f'=f(\frac{v}{v+u_s})$

Towards

$f'=f(\frac{v}{v-u_s})$

$f'=f(\frac{v+u_o}{v})$

Away

$f'=f(\frac{v-u_o}{v})$

$\lambda$ changes
$v$ constant

$\lambda$ constant
$v$ changes

Non-negligible
slit width

Interference pattern modulated by single-slit diffraction envelope

Small angle approximation:

$\sin\theta\approx\theta$

The case found in Formula Booklet

Intensity further from maxima decreases

$\theta=\frac{y}{L}$

$\frac{s}{d}=1.22\frac{\lambda}{b}$
$s=1.22\frac{d\lambda}{b}$

$\frac{s}{d}=\frac{\lambda}{b}$
$s=\frac{d\lambda}{b}$

For two Doppler effects

1⃣ Relative motion between the observer and the source creates frequency change

2⃣

Object is moving
away from observer
🚗📷

Object is moving
towards observer
📷🚗

Incident waves strike observer 🚗 moving away from source 📷

3⃣

Incident waves strike observer 🚗 moving towards source 📷

Decrease in
frequency

Reflected waves come from source 🚗 that is moving towards observer 📷

Reflected waves come from source 🚗 that is moving away from observer 📷

Increase in
frequency

Beam width

$N=\frac{\lambda}{m\Delta\lambda}$

Beam width$=\frac{N}{N_0}\times mm$

Maxima are closer together

Similar to wave at

Similar to reflection of
pulse at free end

Similar to reflection of
pulse at fixed end