POTENTIAL ENERGY AND CONSERVATION OF ENERGY
7.1 Conservative and Nonconservative Forces
Conservative Forces
Nonconservative Forces
- Work done by the force is independent of the particular path
- Net work done by the force on an object moving around any closed path is zero
Example : Gravitational force, Elastic, Electrostatic force
- Work done by the force is dependent on the path taken
- Work done by the force in a closed path is not zero
Example : Friction, Air resistance, Tension in cord, Motor or
rocket propulsion, Push or pull by a person
(a) A system with only conservative forces.
(b) A system with nonconservative forces.
7.2 Potential Energy
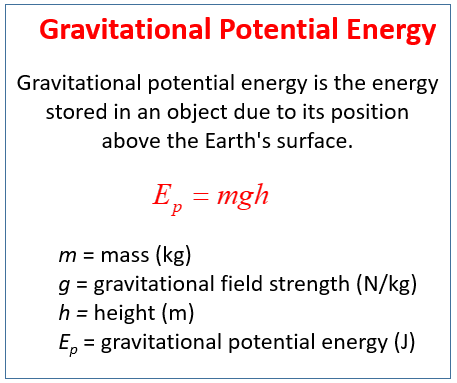
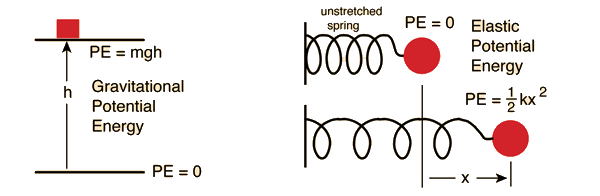
Gravitational Potential Energy
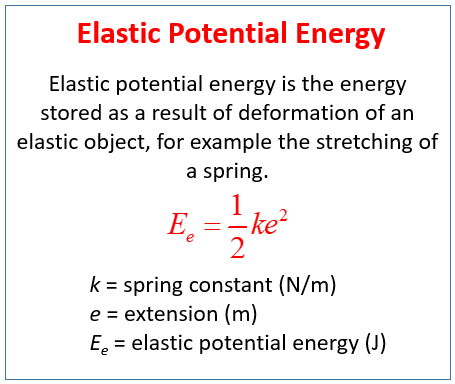
Elastic Potential Energy
Electric Potential Energy
Gravitational Potential Energy
Elastic Potential Energy
7.3 Mechanical Energy and Its Conservation
7.4 Problem Solving Using Conservation of Energy
Principle of Conservation of Mechanical Energy :
the total mechanical energy E remains constant along the path of an object, provided that the net work done by external nonconservative forces is zero
Example :
Gravitational Potential energy vs Kinetic Energy
1/2 mv² + mgh = 1/2mv² + mgh
Kinetic Energy vs Elastic Potential Energy
7.5 The Law of Conservation of Energy 7.6 Energy Conservation with Dissipative Forces:Solving Problem
energy can neither be created nor destroyed, but can only be transformed from one form to another
NONCONSERVATION
CONSERVATION
Forces (friction, heat, chemical energy...) do not conserve mechanical energy, but when these forces taken into account, total energy still conserved
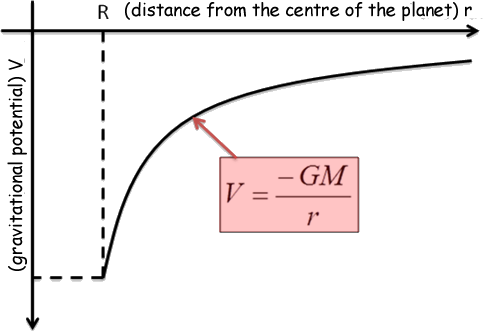
7.7 Gravitational Potential Energy and Escape Velocity
Conservative force
Total Mechanical Energy : E = K + U
Gravitational Potential Energy
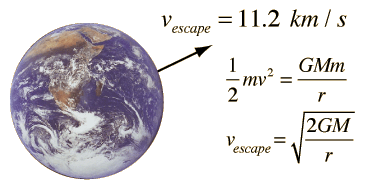
Escape Velocity
the speed that an object needs to be traveling to break free of a planet or moon's gravity well and leave it without further propulsion
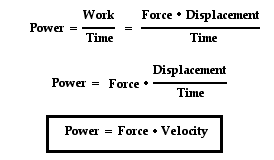
7.8 Power
-the rate at which work is done
Unit : W
scalar quantity
P = Fv
WONG SIEW HAN A20SC0425