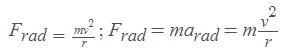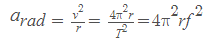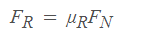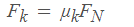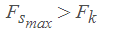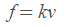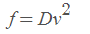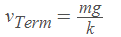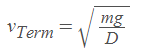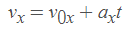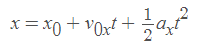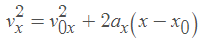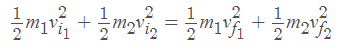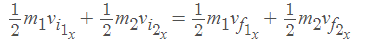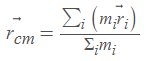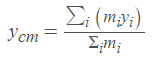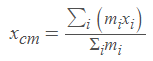Map of Mechanics (Physics)
Kinematics
Forces
Work/Energy
Momentum
Rotational Dynamics
Circular Forces
Centripetal Force
Centrifugal (false/fake/non-existant)
Ciruclar Accelerations
tangential acceleration
radial acceleration
Friction
Kinetic
Static
Rolling
just the apparent force you feel, there isn't actually a force, just inertia of the rotation redirecting your velocity under the radial acceleration.

Fluid Resistance
For slow Speeds in a fluid, the friction on the object is
at higher speeds, the friction becomes proportional to the square of velocity, so the friction becomes
Terminal Speeds
For slow moving objects, the terminal speed is (when Fg = fluid resistance; when mg = kv)
For quicker objects, mg = Dv^2
Kinematic Formulas
acceleration
velocity
distance
initial distance + definite integral of velocity over the interval
initial velocity + definite integral of acceleration over the interval
instantaneous
is the limit as time -> 0
average
is the change in acceleration/velocity/distance over the change in time.
For a,v,or x
Used in problem solving for values - look for the missing variable to find the right formula
Collisions
Impulse
p=mv - p and v are vectors
J = Net force x change in time
J = change in momentum
J = definite integral of net force over time
usually very short times, in milliseconds, with massive net force.
collision classes
Some elasticity
Completely Inelastic
Completely Elastic
Bodies will have the same final velocity
Some kinetic energy lost
kinetic energy is conserved
momentum is conserved
conservation of kinetic energy in elastic collision:
NOTE: the kinetic energy is not a vector! so the x/y components are not conserved, the overall energies of the intial movement and the final movement is conserved!
momentum is conserved
Lost Energy in Collisions:
Lost kinetic energy = initial KE - final KE
KE = 1/2m(p/m)^2
since momentum/mass = speed
the same is true for y values
NOTE: the momentum is conserved only in the components of the vectors, since momentum in non-scalar, you can have two bodies seemingly gain momentum in the y-direction, but its actually just the conservation of momentum working properly.
Center of Mass
the center of mass for a collection of masses is the sum of the bodies respective position times mass, over the sum of the masses
CM is the mass-weighted position of particles
a spinning object, like a tennis racket, will spin around its CM, with the CM following the projectile trajectory
when an object breaks apart in a projectile motion system, the cm will follow the original course, but the split objects will diverge
Total mass x velocity of center of mass = total momentum #
net external force = total mass x acceleration of cm #
Newtons Laws
net force = 0; acceleration = 0
Fa on b = -Fb on a
net force = mass x acceleration
Projectile Motion
Projectile Motion Equations
[on paper]
Coordinates
Velocities
click to edit
click to edit
click to edit
click to edit
Accelerations
ax = const, 0
ay = g
Work
Energy
Kinetic
Potential
W = Scalar product: F * s
W = Fs (force x displacement)
W = Fscos(theta)
K = 1/2mv^2
Work - Energy Theorem:
Wtot = K2 - K1 = deltaK
click to edit
Negative Work
ex: pushing against the direction of motion for a moving object
Variable Work
Integral of the Force function over a definite distance
Springs
Fx = -Kx
the restoration force, has the neg because its opposite its direction of pull, the applied force.
Power
P = W/t
P = dot product: F*v (force times dot product velocity)
Conservation of Energy
on paper
on paper
normal
tension
gravitational
rotating bodies / tangential x, v, and a
click to edit
click to edit
click to edit
Rotational dynamics equations (basically the kinematics but rotating with angles
energy
inertia
parallel axis theorem
kinetic
KE = 1/2 x I x w^2 (half times moment of inertia at pivot point times angular velocity squared)
Ip = Icm + md^2
parallel axis theorem #
Ip = Icm x md^2